--- title: "Fracture mechanics: From the K-factor to damage analysis" description: Fatigue fractures develop depending on their loading situation. Fatigue crack analysis with Ansys SMART allows for inference of this load. image: https://blog.cadfem.net/hubfs/03-Germany/TOPICS/GLOBAL_Blog/Tech%20Artikel%202024/Teaser_Bruchmechanik_640x360.jpg --- Français - Français - Allemand - Anglais [Skip to the main content.](https://blog.cadfem.net/fr/fracture-mechanics-from-the-k-factor-to-damage-analysis#main-content) [![www.cadfem.net](https://blog.cadfem.net/hubfs/01_BASIC/Logos/New%20CADFEM%20Logo/CADFEM_Logo_blue_sRGB_ohne%20Schutzzone%201.svg "www.cadfem.net")](https://blog.cadfem.net/de?c_red=1) [![www.cadfem.net](https://blog.cadfem.net/hubfs/01_BASIC/Logos/New%20CADFEM%20Logo/CADFEM_Logo_blue_sRGB_ohne%20Schutzzone%201.svg "www.cadfem.net")](https://blog.cadfem.net/de?c_red=1) [![](https://blog.cadfem.net/hubfs/Icons/chat.svg) Directcontact](https://blog.cadfem.net/fr/fracture-mechanics-from-the-k-factor-to-damage-analysis#footer-section-contact) - [cadfem.net](https://www.cadfem.net/) - [Cadfem World Conference](https://www.cadfem.net/de/cadfem-informiert/veranstaltungen/cadfem-world-conference.html) - [Ansys Version Dessai](https://www.cadfem.net/fr/nos-solutions/logiciels-de-simulation-ansys-la-gamme-de-produits/ansys-produits-version-dessai.html) - [Français](https://blog.cadfem.net/fr/fracture-mechanics-from-the-k-factor-to-damage-analysis) - [Deutsch](https://blog.cadfem.net/de/bruchmechanik-vom-k-faktor-zur-schadensanalyse) - [English](https://blog.cadfem.net/en/fracture-mechanics-from-the-k-factor-to-damage-analysis) Toggle Menu - [Français](https://blog.cadfem.net/fr/fracture-mechanics-from-the-k-factor-to-damage-analysis) - [Deutsch](https://blog.cadfem.net/de/bruchmechanik-vom-k-faktor-zur-schadensanalyse) - [English](https://blog.cadfem.net/en/fracture-mechanics-from-the-k-factor-to-damage-analysis) Toggle Menu - [cadfem.net](https://www.cadfem.net/) - [Cadfem World Conference](https://www.cadfem.net/de/cadfem-informiert/veranstaltungen/cadfem-world-conference.html) - [Ansys Version Dessai](https://www.cadfem.net/fr/nos-solutions/logiciels-de-simulation-ansys-la-gamme-de-produits/ansys-produits-version-dessai.html) # Fracture mechanics: From the K-factor to damage analysis [Klaus Graf](https://blog.cadfem.net/fr/author/klaus-graf) 18.10.2024 [Structures](https://blog.cadfem.net/fr/tag/structures) [TechArticle](https://blog.cadfem.net/fr/tag/techarticle) LinkedIn Copier le lien We’ve all broken a pretzel stick before and perhaps know that *fracture* caused by bending leads to a 90° angle surface while *fracture* caused by twisting leads to a 45° angle surface. But what load is behind a 35° angle fracture surface? This classic detective work of damage analysis and fracture mechanical simulation with *Ansys Mechanical* is highlighted in this article. ![Hero\_Bruchmechanik\_1093x410](https://blog.cadfem.net/hs-fs/hubfs/03-Germany/TOPICS/GLOBAL_Blog/Tech%20Artikel%202024/Hero_Bruchmechanik_1093x410.jpg?width=1093&height=410&name=Hero_Bruchmechanik_1093x410.jpg) ``` © CADFEM Germany GmbH / Getty Images ``` ## Characterizing Crack Propagation and Fast Rupture Propagation Just as with pretzel sticks, defects in the material, such as pores or inclusions, can occur during production. However, cracks are often also caused by fatigue processes during operational loading. Whether a crack in the component is permissible then depends primarily on whether an existing crack leads to predictable final failure or not. Sticking with the example of the breaking pretzel stick, this is not the case, since the fracture occurs spontaneously upon reaching a critical load (*brittle fracture*). This can also happen with a machine component. The consequences are usually catastrophic, and therefore failure must be avoided at all costs. Fracture mechanics attempts to predict the behavior of a crack under the given loads. It evaluates whether *unstable crack growth* is to be expected at the given crack size and load. If this is not the case, it is also possible to estimate how quickly the crack will continue to grow through stable crack growth, for example due to cyclic loading. During *cyclic crack growth*, the component can still be used safely. However, the end of the service life is reached when the crack has spread so far that either *brittle fracture* is to be expected or the *remaining cross-section* is no longer sufficient to carry the applied loads. In addition to avoiding damage incidents, fracture mechanics can also provide valuable insights when searching for causes of damage. For example, if there is a specific fracture surface orientation, the capabilities of fracture mechanics in Ansys can be used to infer the acting load components. This will be demonstrated in the following analysis. As a geometry, we use a cylinder similar to the shape of pretzel sticks and first use Ansys to verify that the fracture surfaces develop as expected at approximately 45 degrees or normal to the axis in the case of pure torsion and pure tensile stress, respectively. Then we want to find out which load components need to be combined to achieve a 35-degree inclination of the fracture surfaces. ![Tech\_Article\_Bruchmechanik\_Fracture-surface](https://blog.cadfem.net/hs-fs/hubfs/CADFEM%20Blog/Tech%20Artikel%202024/Tech_Article_Bruchmechanik_Fracture-surface.jpg?width=797&height=450&name=Tech_Article_Bruchmechanik_Fracture-surface.jpg) ``` Fracture surface of a Pedal Lever with Fatigue and Rupture Fracture Faces | © By Lokilech, CC BY-SA 3.0, https://commons.wikimedia.org/w/index.php?curid=2239906 ``` ## Understanding Stress Intensity Factors or K-Factors In the context of linear-elastic fracture mechanics, a linear-elastic material behavior is assumed. A crack is modeled as a material separation within the component. At the crack tip, there is intentionally a singularly increasing stress field as shown in the figure below. This determines how the crack behaves further. It would therefore be practical to describe the field using a parameter. In fact, there are three of these parameters *K1*, *K2* and *K3*, each of which indicates the stress increase towards the crack tip depending on the load – the *modes*. *Mode 1*, which is indicated by the index, shows *normal stress* (see figure). *Modes* *2* and *3* characterize *shear stresses*. The parameters calculated and evaluated for the assessment of a crack are called *stress intensity factors* or *K-factors*. In typical cases, these factors generally occur in combination. When *K*-values occur in similar magnitudes, one speaks of “*mixed-mode*” loading, and the three components are combined into an equivalent value according to established hypotheses - similar to converting a stress tensor into an equivalent stress. The *stress intensity factors*, or *K*-factors, change with the loads on the component. Crack growth typically also leads to an increase in the *K*-factors. In a static evaluation, the numerical values must remain below a critical value for the material in the simplest case. In the case of cyclic loading, the range of the stress intensity factors is determined and converted into a *crack growth rate* using empirically determined correlations, allowing for an estimate of the remaining *lifetime* of operation. ![Tech\_Article\_Bruchmechanik\_Singular-Stress-Increase](https://blog.cadfem.net/hs-fs/hubfs/CADFEM%20Blog/Tech%20Artikel%202024/Tech_Article_Bruchmechanik_Singular-Stress-Increase.jpg?width=797&height=584&name=Tech_Article_Bruchmechanik_Singular-Stress-Increase.jpg) ``` Singular Stress Increase toward Crack Tip for Mode 1-Normal Loading. The Increase is described using the K1 parameter | © CADFEM Germany GmbH ``` ## How can the initial crack in a component be modeled using Ansys? To determine the *stress intensity factors* with *Ansys*, an initial crack is required. This is defined using a *Fracture object*. In *Ansys Mechanical*, there is a corresponding crack object for all common crack shapes, with which the crack model can be created with just a few clicks. All that is needed is to set up a local coordinate system for the placement of the crack. The crack is uniquely defined in the crack object along with the required size specifications and mesh parameters. Then, *Ansys Mechanical* builds the crack at the mesh level without geometric modification into a pre-meshed tetrahedra mesh using ‘*Generate All Crack Meshes*’. In this specific example, we consider a smooth cylinder with a diameter of 50 mm. We place a semi-circular crack of 1 mm radius on the cylinder surface. This size is a typical *technical crack* commonly used in fracture mechanics analyses. In this case, the crack surface is defined independently of the applied loading type normal to the shaft axis. Initially, two “classic” load cases will be analyzed, similar to the pretzel stick experiment: tension and torsion. As usual, the load definition is done using the functionalities of *Ansys Mechanical*. However, each load case is analyzed and solved separately in its own analysis system. For the cylinder, a force of 400 kN was applied for the tensile load and a moment of 2.9 kNm for the *torsional load*. With these loads, the nominal comparison load of the uncracked component is approximately 200 MPa in both cases, which is still significantly below potential yield strengths. ``` Definition of a Semi-circular-Crack using the Semi-Elliptical Crack-Object | © CADFEM Germany GmbH ``` ## Assessment of the Stress Intensity Factors of the Modeled Crack During the solution process, the *stress intensity factors* at each node of the crack tip are determined. The calculation requires internal evaluations of an integral around the crack tip. *Ansys* evaluates it several times per node over increasing integration paths – so-called *contours*. The resulting *K*-parameters should be independent of the contour and always deliver the same value. The results of the *contour integrations* are written into the result file and can be evaluated afterwards. To display the *K*-factors along the crack front, the so-called *Fracture Tool* can be used (see figure). The validity of the results is verified by comparing the consistency of the *contour results*. Typically, only the first contour in the immediate vicinity of the crack tip deviates, all other contour results should be close together or at least converge. The following figure shows the graph for *K2* for the torsion load case. The contour results match very well. As can be seen, the units of the *K*-values, such as *MPa*/√mm, are somewhat unusual. This is particularly important to keep in mind when further processing and comparing the numerical values. For the cases studied, there are only significant *K1* values in the *tensile* load case. In the *torsion* load case, both shear components *K2* and *K3* are found. If the crack grows under these conditions, it may develop in another direction and at the same time change the load and possibly also the load modes of the crack. ![Tech\_Article\_Bruchmechanik\_Exemplary-Assessment-Torsion-Case](https://blog.cadfem.net/hs-fs/hubfs/CADFEM%20Blog/Tech%20Artikel%202024/Tech_Article_Bruchmechanik_Exemplary-Assessment-Torsion-Case.jpg?width=797&height=609&name=Tech_Article_Bruchmechanik_Exemplary-Assessment-Torsion-Case.jpg) ``` Exemplary Assessment of the Torsion Case showing Stress Intensity Factor K2 along crack front | © CADFEM Germany GmbH ``` ![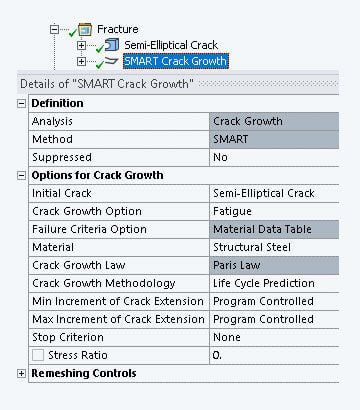Tech\_Article\_Bruchmechanik\_Definition-SMART-Object](https://blog.cadfem.net/hs-fs/hubfs/CADFEM%20Blog/Tech%20Artikel%202024/Tech_Article_Bruchmechanik_Definition-SMART-Object.jpg?width=360&height=410&name=Tech_Article_Bruchmechanik_Definition-SMART-Object.jpg) ``` Definition of a SMART-Object for Analyzing Fatigue Crack Propagation with Ansys Mechanical | © CADFEM Germany GmbH ``` ## Crack Propagation in Ansys is SMART Given a defined initial crack, *Ansys* allows for the analysis of a stepwise crack progression under *static* or *cyclic* loading. The idea behind this is that the ratios of the calculated *K*-values at the crack tip can estimate the direction of further local crack development. Shear loads (*K2* and *K3* values) cause a straight crack to bend in the further course. Together with the magnitude of the *K*-values, a crack progression is then realized by *remeshing* at the crack tip. The functionality is referred to in *Ansys* as *SMART* (“Separating, Morphing, Adaptive and Remeshing Technology”) and is defined with the corresponding object in the mechanical setup. Its definition refers to the initial crack already defined. We continue to use the load cases under consideration as the load for our example. These should each be considered as *pulsating*. This is quantified by the *R*-ratio as in a stress evaluation. We therefore define *R = 0*. In the case of *cyclic fatigue crack growth*, a crack propagation is calculated in each substep, usually using the *Paris-Erdogan* equation. We therefore define a sufficient number of substeps. In our example, 100 for a maximum of 100 crack propagation increments to be analyzed. We deactivate *automatic substepping* as it is not needed for cyclic crack growth. The following figure shows an animation of the crack propagation. ``` Cyclic Crack Propagation in Cylinder with Pulsating Tension Load; Calculated using Ansys SMART | © CADFEM Germany GmbH ``` ## Do pretzel sticks break correctly in Ansys? The crack surfaces formed from the initial crack are presented in the figure below for the two load cases. For *torsional* loading, the axial crack quickly propagates into the expected 45° inclination. Under tensile loading, the crack spreads further normal to the axis. The crack front changes from an original circle to practically a straight shape. The *fracture* images correspond to those expected and ultimately correlate with the observed progressions of the pretzel sticks. So, *Ansys* calculates correctly or, to put it better, our pretzel sticks break correctly! To support a possible damage analysis, we can now ask ourselves which load combination must prevail to achieve a 35° inclination of the crack surface to the axis normal. To do this, we combine *normal* and *torsional* loading in various proportions as cyclic load and calculate the crack progression with *SMART*. We have already done this and were able to iteratively determine a ratio of 55 % of the normal load and 45 % of the *torsional* load to reproduce the damage pattern. Determining the duration at which a crack is spreading can be useful for both preventively and retrospectively in the event of damage. With knowledge of the required material properties, this information falls as a byproduct during the crack progression analysis. The figure shows a comparison of the crack propagation under *tensile*, *torsional* loading, and in our damage examination. The crack grows fastest under *tensile* pulsating load. In our damage case, the crack growth is slightly slowed down compared to pure *tensile* loading. This information can be useful, for example, in dating the crack origin in damage analyses or in deciding on a revision interval in preventive cases. ![Tech\_Article\_Bruchmechanik\_Crack-Growth-Cycle-Counts](https://blog.cadfem.net/hs-fs/hubfs/CADFEM%20Blog/Tech%20Artikel%202024/Tech_Article_Bruchmechanik_Crack-Growth-Cycle-Counts.jpg?width=797&height=453&name=Tech_Article_Bruchmechanik_Crack-Growth-Cycle-Counts.jpg) ``` Crack Growth over Cycle Counts | © CADFEM Germany GmbH ``` ![Tech\_Article\_Bruchmechanik\_Calculated-Crack-States](https://blog.cadfem.net/hs-fs/hubfs/CADFEM%20Blog/Tech%20Artikel%202024/Tech_Article_Bruchmechanik_Calculated-Crack-States.jpg?width=797&height=449&name=Tech_Article_Bruchmechanik_Calculated-Crack-States.jpg) ``` Calculated Crack States for pure Tension, pure Torsion as well as Mixed Cyclic Loading | © CADFEM Germany GmbH ``` ## Outlook Perhaps we could open a small door to the possibilities that *fracture mechanics* has to offer with this contribution. Be assured, there is still a lot more that cannot be addressed within the scope of this article. This includes questions such as how large a crack is actually allowed to grow, as well as the question of the remaining cycle numbers – mentioned in the previous section – which was not shown in detail here. Likewise, the possibilities of crack growth considerations in *Ansys* are far from exhausted with what has been said. For instance, the following questions remain unanswered: What other options for *crack modeling* are available? How can one account for the fact that cracks sometimes close during loading? According to which *hypotheses* is the direction of crack propagation determined? Can *non-proportional load cycles* be analyzed with Ansys? And what options are available to optimize computation times? The CADFEM training “Life with Cracks” offers an in-depth exploration of *fracture mechanics* in *Ansys Mechanical*. We guide you step by step through the fracture mechanics capabilities of *Ansys* *Mechanical* and show you how to conduct a strength assessment according to the “*fracture mechanics*” *FKM guideline* using numerical calculations. ``` Cyclic Crack Propagation under Non-Proportional Loading and Nonlinear Contact Condition of the Cracked Faces | © CADFEM Germany GmbH ``` ### Training on the topic - #### Life with Cracks – Fracture Mechanics Calculation Methods ![bruchmechanik\_cadfem\_seminar\_14417](https://blog.cadfem.net/hs-fs/hubfs/CADFEM%20Blog/Seminar%20Teaser/bruchmechanik_cadfem_seminar_14417.jpg?width=300&height=169&name=bruchmechanik_cadfem_seminar_14417.jpg) In this training, you will learn methods for FE modeling of cracks as well as the most important fracture mechanics parameters. [Info & Registration](https://www.cadfem.net/de/en/shop/professional-development/training-elearning/structural-mechanics/strength/life-with-cracks-fracture-mechanics-calculation-methods-14417.html) - #### Intensive Workshop: Structural Mechanics ![intensive-workshop-structural-mechanics-18975](https://blog.cadfem.net/hs-fs/hubfs/03-Germany/TOPICS/GLOBAL_Blog/Seminar%20Teaser/intensive-workshop-structural-mechanics-18975.jpg?width=300&height=169&name=intensive-workshop-structural-mechanics-18975.jpg) A must-have for all structural mechanics: After this workshop, you will look at your models with different eyes - guaranteed. [Info & Registration](https://www.cadfem.net/de/en/shop/professional-development/intensive-workshops/intensive-workshop-structural-mechanics-18975.html) ### Reference projects on the topic - #### Temperature-influenced thermo-mechanical life cycle analysis of a support structure ![Teaser\_Schnitt-Ofen-Modell](https://blog.cadfem.net/hs-fs/hubfs/Reference%20Projects/Teaser_Schnitt-Ofen-Modell.jpg?width=300&height=169&name=Teaser_Schnitt-Ofen-Modell.jpg) The furnaces of EBNER are developed, among other things, for the heat treatment of semi-finished metal products (steel wires, strips, blanks). Due to the complex thermo-mechanical load, simulations were carried out, which took creep processes into account, in order to evaluate the life cycle prior to the production of a real prototype. [Read the entire reference project](https://www.cadfem.net/en/industries-topics/references/reference/temperature-influenced-thermo-mechanical-life-cycle-analysis-of-a-support-structure.html?c_red=1) - #### Non-linear buckling and load analysis using a simulation wizard ![Liebherr-customer-Crane-engineering-teaser](https://blog.cadfem.net/hs-fs/hubfs/Reference%20Projects/Liebherr-customer-Crane-engineering-teaser.png?width=300&height=169&name=Liebherr-customer-Crane-engineering-teaser.png) At Liebherr, an automated simulation process within Ansys Workbench significantly accelerates and simplifies the non-linear component simulation of thin-walled shell structures in mobile crane construction. The wizard guides the user through all necessary simulation steps. [Read the entire reference project](https://www.cadfem.net/en/industries-topics/references/reference/non-linear-buckling-and-load-analysis-using-a-simulation-wizard.html?c_red=1) - #### Load-optimized implant design ![Teaser\_Project-FHNW](https://blog.cadfem.net/hs-fs/hubfs/Reference%20Projects/Teaser_Project-FHNW.jpg?width=300&height=169&name=Teaser_Project-FHNW.jpg) Design of an optimal patient-specific implant using ANSYS topology optimization: In-silico comparison of acromion levy type ii implants for a patient-specific example. [Read the entire reference project](https://www.cadfem.net/en/industries-topics/references/reference/in-silico-comparison-of-acromion-levy-type-ii-implants-for-a-patient-specific-example.html?c_red=1) ![Portrait\_empty\_Blog](https://blog.cadfem.net/hs-fs/hubfs/TOPICS/GLOBAL_Blog/Tech%20Artikel%202024/Portrait_empty_Blog.jpg?width=400&height=270&name=Portrait_empty_Blog.jpg) ### Author ##### Klaus Graf Engineering Services +49 (0)8092 7005-733 [kgraf@cadfem.de](mailto:kgraf@cadfem.de) ![Portrait\_Blog\_HDonner](https://blog.cadfem.net/hubfs/03-Germany/TOPICS/GLOBAL_Blog/Autoren%20Bilder/Portrait_Blog_HDonner.jpg) ### Editor ##### Dr.-Ing. Hendrik Donner CAE Engineer +49 (0)8092 7005-725 [hdonner@cadfem.de](mailto:hdonner@cadfem.de) ![](https://blog.cadfem.net/hubfs/03-Germany/TOPICS/GLOBAL_Blog/Autoren%20Bilder/Portrait_Blog_MMO-2.jpg) ### Technical Editorial ##### **Dr.-Ing. Marold Moosrainer** Head of Professional Development +49 (0)8092 7005-45 [mmoosrainer@cadfem.de](mailto:mmoosrainer@cadfem.de) Contacter la rédaction Inscription à la newsletter ## Ces articles pourraient également vous intéresser. [![© CADFEM / Adobe Stock](https://blog.cadfem.net/hubfs/topics/GLOBAL_Blog/Blog%20Artikel%202026/2609%20Analog%20Astronaut/Blog_Astronaut_AdobeStock_402438380_640x360jpg.jpg)](https://blog.cadfem.net/fr/astronaute-analogue-simulation-fusees-ansys) [Structures](https://blog.cadfem.net/fr/tag/structures) [Fluide & Thermique](https://blog.cadfem.net/fr/tag/fluide-thermique) [Digitalisation](https://blog.cadfem.net/fr/tag/digitalisation) [Use Case](https://blog.cadfem.net/fr/tag/use-case) #### [Astronaute analogique et simulant simulé](https://blog.cadfem.net/fr/astronaute-analogue-simulation-fusees-ansys) [Alexander Kunz](https://blog.cadfem.net/fr/author/alexander-kunz) | 29.09.2026 [Continuer à lire](https://blog.cadfem.net/fr/astronaute-analogue-simulation-fusees-ansys) [![From the real welding process to digital simulation © CADFEM / Adobe Stock. Image AI-generated.](https://blog.cadfem.net/hubfs/topics/GLOBAL_Blog/Blog%20Artikel%202026/2608%20TechArticle%20WeldTool/26_09_SEH_Welding%20Toolbox_Teaser_640x360.jpg)](https://blog.cadfem.net/fr/welding-process-simulation-made-easy) [Structures](https://blog.cadfem.net/fr/tag/structures) [Fluide & Thermique](https://blog.cadfem.net/fr/tag/fluide-thermique) [TechArticle](https://blog.cadfem.net/fr/tag/techarticle) #### [Welding Process Simulation Made Easy!](https://blog.cadfem.net/fr/welding-process-simulation-made-easy) [Sebastian Hoffmann](https://blog.cadfem.net/fr/author/sebastian-hoffmann) | 22.09.2026 [Continuer à lire](https://blog.cadfem.net/fr/welding-process-simulation-made-easy) [![](https://blog.cadfem.net/hubfs/topics/GLOBAL_Blog/Blog%20Artikel%202026/2608_EMFC/26_07_HB_Verhaltensmodelle_Teaser_640x360.jpg)](https://blog.cadfem.net/fr/emfc-scale-how-simulation-makes-temperature-drift-visible) [Structures](https://blog.cadfem.net/fr/tag/structures) [Fluide & Thermique](https://blog.cadfem.net/fr/tag/fluide-thermique) [Eléctronique](https://blog.cadfem.net/fr/tag/eléctronique) [TechArticle](https://blog.cadfem.net/fr/tag/techarticle) #### [EMFC Scale: How Simulation Makes Temperature Drift Visible](https://blog.cadfem.net/fr/emfc-scale-how-simulation-makes-temperature-drift-visible) [Hanna Baumgartl](https://blog.cadfem.net/fr/author/hanna-baumgartl) | 10.09.2026 [Continuer à lire](https://blog.cadfem.net/fr/emfc-scale-how-simulation-makes-temperature-drift-visible) [![](https://blog.cadfem.net/hubfs/01_BASIC/Logos/New%20CADFEM%20Logo/CADFEM_Logo_blue_sRGB_ohne%20Schutzzone%201.svg)](https://www.cadfem.net/de/en/home.html) - [Mentions légales](https://www.cadfem.net/fr/mentions-legales) - [CGV](https://www.cadfem.net/fr/agb) - [Politique de confidentialité](https://www.cadfem.net/fr/politique-de-confidentialite) - [Conditions d'utilisation](https://www.cadfem.net/fr/conditions-dutilisation) - [Supprimer et gérer les cookies](https://www.cadfem.net/fr/note-de-cookie) © 2026 CADFEM Germany GmbH *Return to Top* ```json { "@context" : "https://schema.org", "@type" : "BlogPosting", "author" : { "@type" : "Person", "name" : "Klaus Graf", "url" : "https://blog.cadfem.net/fr/author/klaus-graf" }, "dateModified" : "2026-08-11T07:50:44.188Z", "datePublished" : "2024-10-18T09:25:07.000Z", "headline" : "Fracture mechanics: From the K-factor to damage analysis", "image" : [ "https://blog.cadfem.net/hubfs/03-Germany/TOPICS/GLOBAL_Blog/Tech%20Artikel%202024/Teaser_Bruchmechanik_640x360.jpg" ], "mainEntityOfPage" : { "@id" : "https://blog.cadfem.net/fr/fracture-mechanics-from-the-k-factor-to-damage-analysis", "@type" : "WebPage" }, "publisher" : { "@type" : "Organization", "logo" : { "@type" : "ImageObject", "url" : "https://blog.cadfem.net/hubfs/CADFEM_Logo_blue_sRGB_ohne%20Schutzzone%201-1.png" }, "name" : "CADFEM Germany GmbH" } } ```